More on the running track
4 posters
Page 1 of 1
 More on the running track
More on the running track
Paper: milesmathis.com/track.pdf
This was really interesting, talking about the human body elasticity, leaning while running and also there was a mainstream article about why on the curves we should slow down that would award any high school student the lowest mark.
I propose an experiment, but first an introduction. The human body has that advantage in curves Mathis talked about, but mechanical objects usually don't. We can take any robot (maybe a sphero) with a low maximum speed, and make it run at that maximum speed along a 200 m track in various lanes. The speed is constant, the sphero according to Mathis theory will take different times then, fewer on line 8 and more on line 1.
This was really interesting, talking about the human body elasticity, leaning while running and also there was a mainstream article about why on the curves we should slow down that would award any high school student the lowest mark.
I propose an experiment, but first an introduction. The human body has that advantage in curves Mathis talked about, but mechanical objects usually don't. We can take any robot (maybe a sphero) with a low maximum speed, and make it run at that maximum speed along a 200 m track in various lanes. The speed is constant, the sphero according to Mathis theory will take different times then, fewer on line 8 and more on line 1.
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
Ciaolo,
Your challenge, sending fixed speed spheros (what’s a sphero?) along each lane of a 200m track sounds easy enough.
It’s even easier to just calculate the ‘true’ lane distances, using pi=4 for the curves. For starters, find some track dimensions along with specific race start/stop points, and try it. Since I'm trying to learn R script, I’ll be happy to help. It would be great to have math examples to highlight Miles' papers.
.
Your challenge, sending fixed speed spheros (what’s a sphero?) along each lane of a 200m track sounds easy enough.
It’s even easier to just calculate the ‘true’ lane distances, using pi=4 for the curves. For starters, find some track dimensions along with specific race start/stop points, and try it. Since I'm trying to learn R script, I’ll be happy to help. It would be great to have math examples to highlight Miles' papers.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
A sphero is a remotely controlled toy robot that has the shape of a sphere and some motors inside that make it rotate to move, like the BB-8 of the newest Star Wars movie.
Another experiment is doing a lap with marking shoes and calculate the average distance of the marks in the straights and in the curves. According to mainstream, the average distance of the marks on the curves must be smaller than the one for the straights. According to Mathis theory it should be greater.
Of course this should be done by a marathon athlete so that they can assure a constant speed.
Another experiment is doing a lap with marking shoes and calculate the average distance of the marks in the straights and in the curves. According to mainstream, the average distance of the marks on the curves must be smaller than the one for the straights. According to Mathis theory it should be greater.
Of course this should be done by a marathon athlete so that they can assure a constant speed.
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
Thanks to the government of Western Australia, Dept. of Sport and Recreation.
http://www.dsr.wa.gov.au/support-and-advice/facility-management/developing-facilities/dimensions-guide/sport-specific-dimensions/athletics-track-events
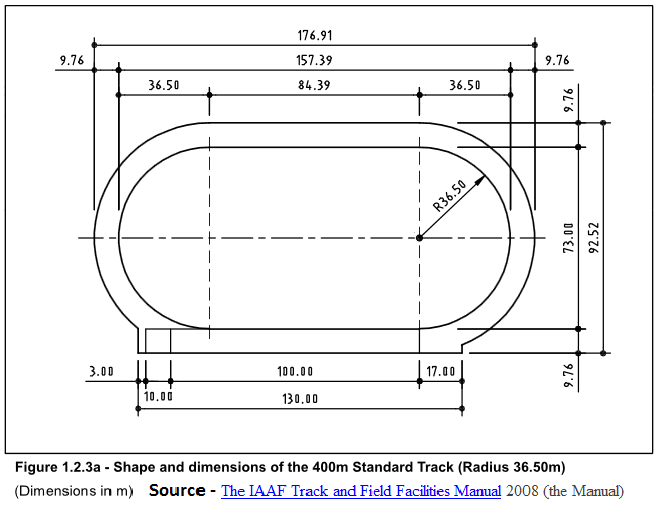
I'm adding lanes now.
.
http://www.dsr.wa.gov.au/support-and-advice/facility-management/developing-facilities/dimensions-guide/sport-specific-dimensions/athletics-track-events

I'm adding lanes now.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
Wow, I hope Mathis reads this forum 
Anyway, machines and the likes aren't dynamic like the human body as they run curves, but there is one exception.
Motorbikes have suspensions, and they lean on turns. The principle Mathis talked aobut in the OP paper applies to them as well, does it?
In races usually vehicles try to do the most wide curve in turns. They do that so they can lose the least amount of speed possible when doing turns. But since that path is also the one that contains the longest curve segment and motorbikes can gain speed on any curve (this is still to see), shouldn't exist another path that for motorbikes only is better than the usual one? Maybe the shortest curve possible that has a certain minimum radius based on the motorbike length/distance between wheels/suspensions.
But maybe I'm mistaken
Anyway, machines and the likes aren't dynamic like the human body as they run curves, but there is one exception.
Motorbikes have suspensions, and they lean on turns. The principle Mathis talked aobut in the OP paper applies to them as well, does it?
In races usually vehicles try to do the most wide curve in turns. They do that so they can lose the least amount of speed possible when doing turns. But since that path is also the one that contains the longest curve segment and motorbikes can gain speed on any curve (this is still to see), shouldn't exist another path that for motorbikes only is better than the usual one? Maybe the shortest curve possible that has a certain minimum radius based on the motorbike length/distance between wheels/suspensions.
But maybe I'm mistaken
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
.

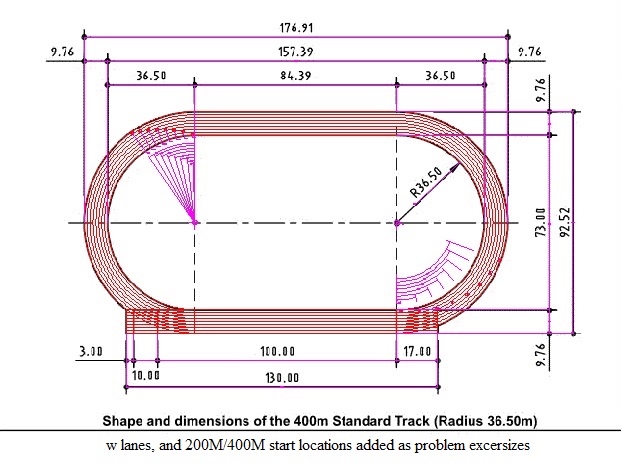
I've added the lanes (red) and 200m starting lines (blue).
I'm showing how the angles are determined, measured to the left of the vertical blue line of the left semicircle.

I'm surprised by the constant total distance - 231.59m. Any comments? I'll carry on with the 400m.
.

I've added the lanes (red) and 200m starting lines (blue).
I'm showing how the angles are determined, measured to the left of the vertical blue line of the left semicircle.

I'm surprised by the constant total distance - 231.59m. Any comments? I'll carry on with the 400m.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
Wow I thought you made some mistakes but I did slightly different calculations and the results are the same.
It can't be.
Will look better into it and find out a solution!
EDIT: yes, the solution is this:
the ratio between the 3.14 circumference and the 4 circumference is the same for all the lanes, so all of them are long exactly the same.
So, the pi=4 must be proven in some other way. If we do that sphero experiment we will still notice that it takes more time than it should. Also, I expect that any simulation should be able to come to the same conclusion.
EDIT 2: I really hope Mathis is reading this to see his thought about it in the next papers xD
It can't be.
Will look better into it and find out a solution!
EDIT: yes, the solution is this:
the ratio between the 3.14 circumference and the 4 circumference is the same for all the lanes, so all of them are long exactly the same.
So, the pi=4 must be proven in some other way. If we do that sphero experiment we will still notice that it takes more time than it should. Also, I expect that any simulation should be able to come to the same conclusion.
EDIT 2: I really hope Mathis is reading this to see his thought about it in the next papers xD
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
I just found a comment about building a 200m straight dedicated track. It was 12 years ago after Atlanta olympics (wow the Internet).
This comment was really interesting:
Also is so unsettling to see that thread full of insults on a topic like this...
(link is http://rec.sport.olympics.narkive.com/aprUprfB/why-no-straight-track-for-100-200-m )
And, as final touch to this awesome post (if I say so myself) let's do this experiment:
Take a stopwatch, watch a video of a 100m relay and see how the various athletes that run mostly on curves perform. And prepare to be amazed
This comment was really interesting:
That was so frustrating! I had this friend who insisted that MichaelThat's a good idea actually. If they did that in Atlanta, then there wouldn't
have been all those idiots saying "Wow, if you divide Michael Johnson's 200m
time in half, then the time you get is lower than the world record for the
100m!!!"
Johnson's first 100 was faster than the second 100 (because as everyone
knows, sprinters decelerate in the second half), and that the first 100 time
would have won gold in the 100m. He wouldn't budge on either of his beliefs.
Also is so unsettling to see that thread full of insults on a topic like this...
(link is http://rec.sport.olympics.narkive.com/aprUprfB/why-no-straight-track-for-100-200-m )
And, as final touch to this awesome post (if I say so myself) let's do this experiment:
Take a stopwatch, watch a video of a 100m relay and see how the various athletes that run mostly on curves perform. And prepare to be amazed
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
Ok, The surprise may be gone, but here are my numbers.

The only physical difference present, (besides vantage of rivals) seems to be that each runner is subject to different lane curvatures, the forces associated with that curvature, and where one starts in that curvature.
I agree that forces need to be looked at next.
.
P.S. Note The 400M col header starting with "115.61" should be changed to "231.32".
Last edited by LongtimeAirman on Mon Sep 19, 2016 8:43 pm; edited 2 times in total (Reason for editing : Added PS and changed ending the of the sentence ending n curvature.)
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
Miles sent me a link to this video:
.
A simpler experiment to show kinematic pi equals 4.
.
A simpler experiment to show kinematic pi equals 4.
Last edited by Nevyn on Wed Sep 21, 2016 7:40 pm; edited 1 time in total (Reason for editing : Fixed styling to show video in-place)
 Re: More on the running track
Re: More on the running track
Nevyn wrote:Miles sent me a link to this video: https://m.youtube.com/watch?v=7GuRnc92IZ0&feature=youtu.be.
A simpler experiment to show kinematic pi equals 4.
Sorry for the lack of style, stuck on mobile.
Nice video Nevyn. Very direct and clear cut with a real-life demonstration.
 Re: More on the running track
Re: More on the running track
I would like to know the velocity of each ball as it emerges from the end. Are they different? The same? If different, what is the relationship between them?
 Re: More on the running track
Re: More on the running track
.
Nice video.
I had doubts:
1) what about friction between the steel ball bearing and the internal surface of the plastic tubing - but the clear and immediate slowdown of the ball entering the loop, and the simultaneous exits answers that.
2) the steel ball traveling the circle cannot remain at the lowest point (or line) of the tube. The ball's path is on the slope, or ramp and therefore the path is longer. I gather the difference is too small to affect the outcome.
Nevyn wrote: I would like to know the velocity of each ball as it emerges from the end. Are they different? The same? If different, what is the relationship between them?
Good experimental recommendation, include a second straight ramp after the loop to allow velocity comparisons, beginning and end. I think we'll find the four straight velocities are the same (ignoring friction), but of course you're correct to ask. We cannot see forces, but we can see velocities.
.
Nice video.
I had doubts:
1) what about friction between the steel ball bearing and the internal surface of the plastic tubing - but the clear and immediate slowdown of the ball entering the loop, and the simultaneous exits answers that.
2) the steel ball traveling the circle cannot remain at the lowest point (or line) of the tube. The ball's path is on the slope, or ramp and therefore the path is longer. I gather the difference is too small to affect the outcome.
Nevyn wrote: I would like to know the velocity of each ball as it emerges from the end. Are they different? The same? If different, what is the relationship between them?
Good experimental recommendation, include a second straight ramp after the loop to allow velocity comparisons, beginning and end. I think we'll find the four straight velocities are the same (ignoring friction), but of course you're correct to ask. We cannot see forces, but we can see velocities.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
.
I complemented, then asked the poster to consider adding a final straight to compare before and after velocities, then realized that the plastic tubing cannot cross itself while staying on a single flat surface, z=0. Any up or down slopes will mess with the simplicity.
My mind turns to spirals. Say I have 100m of rope tied to two trees 10m apart. To take up the slack, I’ll lay the rope into a coil. In order to maintain the existing angular relationship between the rope’s end’s, I’ll have to introduce a full 360deg twist for each additional loop. That twist is an additional orthogonal rotation. A proper way to lay it flat again is to unspool it as from a horizontal axis.
My big insight so far, (it may be fleeting): With no rolling slippage, the ball is spinning along the internal tubing surface exactly as in the definition of a centroid.
Please correct me if you see fit. There is no reason to expect a change in velocity - before and after; instead, we would expect to see a spin reaction to that 360deg twisting with each loop.
P.S. Make that a 180deg twist for each loop.
.
I complemented, then asked the poster to consider adding a final straight to compare before and after velocities, then realized that the plastic tubing cannot cross itself while staying on a single flat surface, z=0. Any up or down slopes will mess with the simplicity.
My mind turns to spirals. Say I have 100m of rope tied to two trees 10m apart. To take up the slack, I’ll lay the rope into a coil. In order to maintain the existing angular relationship between the rope’s end’s, I’ll have to introduce a full 360deg twist for each additional loop. That twist is an additional orthogonal rotation. A proper way to lay it flat again is to unspool it as from a horizontal axis.
My big insight so far, (it may be fleeting): With no rolling slippage, the ball is spinning along the internal tubing surface exactly as in the definition of a centroid.
Please correct me if you see fit. There is no reason to expect a change in velocity - before and after; instead, we would expect to see a spin reaction to that 360deg twisting with each loop.
P.S. Make that a 180deg twist for each loop.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
The velocities are different because the circular path is shorter. The difference should be the relationship between pi and 4.
Both balls have friction with the tube so I think we can rule that out as close enough.
The curved path does change the direction so it does slow it down but that is the point of the experiment. Curved motion requires 2 velocities and the tube is providing the 2nd velocity.
The guy in the video used to post to the EU forum as Steve-o. Can anyone get in contact with him? He would be a good addition to our little team here. A friendly invite can't hurt.
Both balls have friction with the tube so I think we can rule that out as close enough.
The curved path does change the direction so it does slow it down but that is the point of the experiment. Curved motion requires 2 velocities and the tube is providing the 2nd velocity.
The guy in the video used to post to the EU forum as Steve-o. Can anyone get in contact with him? He would be a good addition to our little team here. A friendly invite can't hurt.
 Re: More on the running track
Re: More on the running track
.
Velocity after, Vf, is the velocity of the sphere exiting the loop. Ignoring friction, I believe Vi = Vf.
The velocity in the loop, Vl, certainly appears slower than the straight by what could be pi/4. As you say, there are two velocities involved. I think there is just one velocity, the difference is the fact that the physical distance for an object in a kinetic looping motion is 4/pi longer
.
P.S. Just to clarify. In my opinion, there aren't two velocities in the loop. The single kinetic velocity must, however, be resolved into two orthogonal velocity components. I believe these are the two velocities you are referring to. The cycloid reveals the distance in a loop is 4/pi longer than a straight section. For the straight section the second orthogonal velocity component is zero.
.
Velocity before, Vi, is the speed at the bottom of the straight ramp before the ball enters the loop.The velocities are different because the circular path is shorter. The difference should be the relationship between pi and 4.
Velocity after, Vf, is the velocity of the sphere exiting the loop. Ignoring friction, I believe Vi = Vf.
The velocity in the loop, Vl, certainly appears slower than the straight by what could be pi/4. As you say, there are two velocities involved. I think there is just one velocity, the difference is the fact that the physical distance for an object in a kinetic looping motion is 4/pi longer
I think the balls are maintaining a rolling contact with the tube. Nothing dramatic.Both balls have friction with the tube so I think we can rule that out as close enough.
Agreed. I ask that you consider my twisting rope idea. I call it an orthogonal spin because that’s what it appears to be.The curved path does change the direction so it does slow it down but that is the point of the experiment. Curved motion requires 2 velocities and the tube is providing the 2nd velocity.
Yes! I invited him.The guy in the video used to post to the EU forum as Steve-o. Can anyone get in contact with him? He would be a good addition to our little team here. A friendly invite can't hurt.
.
P.S. Just to clarify. In my opinion, there aren't two velocities in the loop. The single kinetic velocity must, however, be resolved into two orthogonal velocity components. I believe these are the two velocities you are referring to. The cycloid reveals the distance in a loop is 4/pi longer than a straight section. For the straight section the second orthogonal velocity component is zero.
.
Last edited by LongtimeAirman on Wed Sep 21, 2016 10:16 pm; edited 2 times in total (Reason for editing : Added PS)
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
What if the tubes were pneumatic tubes with the same vacuum pressure. With the balls floating in the middle of the tubes and pulled at the same pressure (equal Bernoulli effect). Would the outcome be any different?
 Re: More on the running track
Re: More on the running track
.
The single loop velocity in the video is comparable to orbital velocity. Orbital velocity, Miles explains, is a misnomer since orbits curve. Every dt the velocity changes direction. Orbital velocities are therefore accelerations. The apparent orbital velocity must be replaced with its component orthogonal velocities in order to understand the mechanics.
We are beginning to appreciate the differences between curved and straight motion. The cycloid shows how curves are 4/pi longer than straights. The tube constrains the ball to curved motion. The pneumatic example seems to remove a contact, so where is its cycloid? The contact isn't present in orbits either yet the outcome is the same. The ratio is always present in curved motion itself.
.
What if the tubes were pneumatic tubes with the same vacuum pressure. With the balls floating in the middle of the tubes and pulled at the same pressure (equal Bernoulli effect). Would the outcome be any different?
The single loop velocity in the video is comparable to orbital velocity. Orbital velocity, Miles explains, is a misnomer since orbits curve. Every dt the velocity changes direction. Orbital velocities are therefore accelerations. The apparent orbital velocity must be replaced with its component orthogonal velocities in order to understand the mechanics.
We are beginning to appreciate the differences between curved and straight motion. The cycloid shows how curves are 4/pi longer than straights. The tube constrains the ball to curved motion. The pneumatic example seems to remove a contact, so where is its cycloid? The contact isn't present in orbits either yet the outcome is the same. The ratio is always present in curved motion itself.
.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
.

Below is the R script I put together for the 400M Track. It took me longer than I imagined, a real series of puzzles. R seems much more difficult that CAD. All the comment obscured lines plots the track outline in far less time. All the plot lines active gives the above. The code is ugly, it needs loops, but all the coordinates are in there, well, the angles in the tables above (previous posts) are replaced with x and y coordinates in the plot lines.
My first shot at contacting Steve is a miss. If anyone else can help I would certainly appreciate it. I've been thinking about his experiment. He cannot include a straight at the end of the circle since the tube is blocking itself. I figure an s-loop is as good as a circle, so we can have a 1) ramp and straight track; 2)ramp and circle; or 3) ramp, s-curve, straight.Yes! I invited him.The guy in the video used to post to the EU forum as Steve-o. Can anyone get in contact with him? He would be a good addition to our little team here. A friendly invite can't hurt.

Below is the R script I put together for the 400M Track. It took me longer than I imagined, a real series of puzzles. R seems much more difficult that CAD. All the comment obscured lines plots the track outline in far less time. All the plot lines active gives the above. The code is ugly, it needs loops, but all the coordinates are in there, well, the angles in the tables above (previous posts) are replaced with x and y coordinates in the plot lines.
- Code:
library(ggplot2)
ggplot(data=data.frame(x=1,y=1),aes(x,y))+
###Plots a 400M Track
###topleft quarter circle:
geom_path(data=data.frame(x=c(28.61+(-46260:(-1)/1000),28.61),y=c(46.26+sqrt((46.26)^2-c(-46260:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-45040:(-1)/1000),28.61),y=c(46.26+sqrt((45.04)^2-c(-45040:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-43820:(-1)/1000),28.61),y=c(46.26+sqrt((43.82)^2-c(-43820:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-42600:(-1)/1000),28.61),y=c(46.26+sqrt((42.6)^2-c(-42600:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-41380:(-1)/1000),28.61),y=c(46.26+sqrt((41.38)^2-c(-41380:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-40160:(-1)/1000),28.61),y=c(46.26+sqrt((40.16)^2-c(-40160:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-38940:(-1)/1000),28.61),y=c(46.26+sqrt((38.94)^2-c(-38940:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-37720:(-1)/1000),28.61),y=c(46.26+sqrt((37.72)^2-c(-37720:(-1)/1000,0)^2))),aes(x=x,y=y))+
geom_path(data=data.frame(x=c(28.61+(-36500:(-1)/1000),28.61),y=c(46.26+sqrt((36.5)^2-c(-36500:(-1)/1000,0)^2))),aes(x=x,y=y))+
###200Start
#geom_path(data=data.frame(x=c(-607:(-1)/1000+2.4641,1:607/1000+2.4641,607:1/1000+2.4641,-1:-607/1000+2.4641),y=c(c(83.6932+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(83.6932-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+5.7701,1:607/1000+5.7701,607:1/1000+5.7701,-1:-607/1000+5.7701),y=c(c(84.3841+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(84.3841-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+9.1787,1:607/1000+9.1787,607:1/1000+9.1787,-1:-607/1000+9.1787),y=c(c(84.8664+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(84.8664-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+12.6976,1:607/1000+12.6976,607:1/1000+12.6976,-1:-607/1000+12.6976),y=c(c(85.1279+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(85.1279-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+16.3182,1:607/1000+16.3182,607:1/1000+16.3182,-1:-607/1000+16.3182),y=c(c(85.1404+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(85.1404-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+20.0260,1:607/1000+20.0260,607:1/1000+20.0260,-1:-607/1000+20.0260),y=c(c(84.8725+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(84.8725-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+23.8033,1:607/1000+23.8033,607:1/1000+23.8033,-1:-607/1000+23.8033),y=c(c(84.2904+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(84.2904-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+27.6237,1:607/1000+27.6237,607:1/1000+27.6237,-1:-607/1000+27.6237),y=c(c(83.3574+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(83.3574-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
###bottomleft quarter circle:
geom_path(data=data.frame(x=c(28.61+(-46260:(-1)/1000),28.61),y=c(46.26-sqrt((46.26)^2-c(-46260:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-45040:(-1)/1000),28.61),y=c(46.26-sqrt((45.04)^2-c(-45040:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-43820:(-1)/1000),28.61),y=c(46.26-sqrt((43.82)^2-c(-43820:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-42600:(-1)/1000),28.61),y=c(46.26-sqrt((42.6)^2-c(-42600:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-41380:(-1)/1000),28.61),y=c(46.26-sqrt((41.38)^2-c(-41380:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-40160:(-1)/1000),28.61),y=c(46.26-sqrt((40.16)^2-c(-40160:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-38940:(-1)/1000),28.61),y=c(46.26-sqrt((38.94)^2-c(-38940:(-1)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(28.61+(-37720:(-1)/1000),28.61),y=c(46.26-sqrt((37.72)^2-c(-37720:(-1)/1000,0)^2))),aes(x=x,y=y))+
geom_path(data=data.frame(x=c(28.61+(-36500:(-1)/1000),28.61),y=c(46.26-sqrt((36.5)^2-c(-36500:(-1)/1000,0)^2))),aes(x=x,y=y))+
###topright quarter circle:
geom_path(data=data.frame(x=c(113+(46260:(0)/1000),113),y=c(46.26+sqrt((46.26)^2-c(46260:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(45040:(0)/1000),113),y=c(46.26+sqrt((45.04)^2-c(45040:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(43820:(0)/1000),113),y=c(46.26+sqrt((43.82)^2-c(43820:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(42600:(0)/1000),113),y=c(46.26+sqrt((42.60)^2-c(42600:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(41380:(0)/1000),113),y=c(46.26+sqrt((41.38)^2-c(41380:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(40160:(0)/1000),113),y=c(46.26+sqrt((40.16)^2-c(40160:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(38940:(0)/1000),113),y=c(46.26+sqrt((38.94)^2-c(38940:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(37720:(0)/1000),113),y=c(46.26+sqrt((37.72)^2-c(37720:(0)/1000,0)^2))),aes(x=x,y=y))+
geom_path(data=data.frame(x=c(113+(36500:(0)/1000),113),y=c(46.26+sqrt((36.50)^2-c(36500:(0)/1000,0)^2))),aes(x=x,y=y))+
###bottomright quarter circle:
geom_path(data=data.frame(x=c(113+(46260:(0)/1000),113),y=c(46.26-sqrt((46.26)^2-c(46260:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(45040:(0)/1000),113),y=c(46.26-sqrt((45.04)^2-c(45040:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(43820:(0)/1000),113),y=c(46.26-sqrt((43.82)^2-c(43820:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(42600:(0)/1000),113),y=c(46.26-sqrt((42.60)^2-c(42600:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(41380:(0)/1000),113),y=c(46.26-sqrt((41.38)^2-c(41380:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(40160:(0)/1000),113),y=c(46.26-sqrt((40.16)^2-c(40160:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(38940:(0)/1000),113),y=c(46.26-sqrt((38.94)^2-c(38940:(0)/1000,0)^2))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(113+(37720:(0)/1000),113),y=c(46.26-sqrt((37.72)^2-c(37720:(0)/1000,0)^2))),aes(x=x,y=y))+
geom_path(data=data.frame(x=c(113+(36500:(0)/1000),113),y=c(46.26-sqrt((36.50)^2-c(36500:(0)/1000,0)^2))),aes(x=x,y=y))+
###400Start
#geom_path(data=data.frame(x=c(-607:(-1)/1000+155.8343,1:607/1000+155.8343,607:1/1000+155.8343,-1:-607/1000+155.8343),y=c(c(30.4462+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(30.4462-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+152.1487,1:607/1000+152.1487,607:1/1000+152.1487,-1:-607/1000+152.1487),y=c(c(25.2245+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(25.2245-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+147.6647,1:607/1000+147.6647,607:1/1000+147.6647,-1:-607/1000+147.6647),y=c(c(20.4455+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(20.4455-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+142.3906,1:607/1000+142.3906,607:1/1000+142.3906,-1:-607/1000+142.3906),y=c(c(16.2582+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(16.2582-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+136.3659,1:607/1000+136.3659,607:1/1000+136.3659,-1:-607/1000+136.3659),y=c(c(12.8412+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(12.8412-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+129.6752,1:607/1000+129.6752,607:1/1000+129.6752,-1:-607/1000+129.6752),y=c(c(10.3915+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(10.3915-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+122.4440,1:607/1000+122.4440,607:1/1000+122.4440,-1:-607/1000+122.4440),y=c(c(9.1086+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(9.1086-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
#geom_path(data=data.frame(x=c(-607:(-1)/1000+114.8294,1:607/1000+114.8294,607:1/1000+114.8294,-1:-607/1000+114.8294),y=c(c(9.1946+sqrt(0.607^2-c(-607:(-1)/1000,1:607/1000)^2)),c(9.1946-sqrt(0.607^2-c(607:1/1000,-1:-607/1000)^2)))),aes(x=x,y=y))+
###130 straight:
geom_path(data=data.frame(x=c(0,130),y=c(0,0)))+
#geom_path(data=data.frame(x=c(0,130),y=c(1.2200,1.2200)))+
#geom_path(data=data.frame(x=c(0,130),y=c(2.4400,2.4400)))+
#geom_path(data=data.frame(x=c(0,130),y=c(3.6600,3.6600)))+
#geom_path(data=data.frame(x=c(0,130),y=c(4.8800,4.8800)))+
#geom_path(data=data.frame(x=c(0,130),y=c(6.1000,6.1000)))+
#geom_path(data=data.frame(x=c(0,130),y=c(7.3200,7.3200)))+
#geom_path(data=data.frame(x=c(0,130),y=c(8.5400,8.5400)))+
geom_path(data=data.frame(x=c(0,130),y=c(9.7600,9.7600)))+
### 130 distances:
geom_path(data=data.frame(x=c(0,0),y=c(0,9.7600)))+
geom_path(data=data.frame(x=c(3,3),y=c(0,9.7600)))+
geom_path(data=data.frame(x=c(13,13),y=c(0,9.7600)))+
geom_path(data=data.frame(x=c(113,113),y=c(0,9.7600)))+
geom_path(data=data.frame(x=c(130,130),y=c(0,9.7600)))+
### Back straight:
geom_path(data=data.frame(x=c(28.61,113),y=c(92.5200,92.52)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(91.3000,91.3000)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(90.0800,90.0800)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(88.8600,88.8600)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(87.6400,87.6400)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(86.4200,86.4200)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(85.2000,85.2000)))+
#geom_path(data=data.frame(x=c(28.61,113),y=c(83.9800,83.9800)))+
geom_path(data=data.frame(x=c(28.61,113),y=c(82.7600,82.7600)))+
###fix aspect ratio to 1:1
coord_fixed()
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Re: More on the running track
Re: More on the running track
Why doing an S-shaped path when you can do the experiment with a C-shaped path?
Ciaolo- Posts : 143
Join date : 2016-09-08
 Re: More on the running track
Re: More on the running track
.
Here's what I'm thinking - running with Nevyn's comment.

.
Here's what I'm thinking - running with Nevyn's comment.

.
LongtimeAirman- Admin
- Posts : 2078
Join date : 2014-08-10
 Similar topics
Similar topics» PI NINE - Pi experiment with two edge track
» On the track of unconventional superconductivity, researchers are charting unknown territory
» On the track of unconventional superconductivity, researchers are charting unknown territory
Page 1 of 1
Permissions in this forum:
You cannot reply to topics in this forum